How can you prove it? With maths!

How do you prove something? What even is proof?

In science, the word ‘proof’ is used rarely and with great care. Scientists accept that the natural world is full of surprises, and what appears to be true may have exceptions.

An 18th century engraving of Raphael’s School of Athens. The painting depicts famous artists, philosophers, mathematicians and scientists. In the centre are Plato (modelled by Leonardo da Vinci) and on his right, Aristotle. Diogenes is slumped on the steps below their feet. Leaning on a working table is Heraclites. Seated left-foreground is Pythagoras. 

In the law courts, proof often includes a caveat, such as ‘on the balance of probabilities’ for civil matters, and ‘beyond reasonable doubt’ for criminal cases.

But to mathematicians like the University of Melbourne’s Dr Nick Beaton, Professor Jan de Gier and Professor Tony Guttmann, ‘beyond reasonable doubt’ simply isn’t good enough. To them, a mathematical proof is ‘beyond any doubt’ – and it is a thing of beauty.

Consider Pythagoras’ Theorem.

We all learn in school that the square of the longest side of a right-angled triangle is the sum of the squares of the two other sides. You can test this with a piece of paper, a ruler and a calculator, and you’ll see that it’s true.

You could do this for a thousand triangles and you’ll see that it’s true for every one of those thousand triangles.

But does Pythagoras’ Theorem work for every possible right-angled triangle?

You can’t measure every right-angled triangle that exists, so the ruler and calculator method cannot definitively prove Pythagoras right.

“You do a lot of simulations and you observe a certain thing numerically, and if you observe that over and over and over again you would think that that’s probably always the case, or it’s true,” says Dr Nick Beaton.

“But that’s not quite the same as having a mathematical proof where you can actually logically show that a certain thing always happens at certain values of the parameters.”

A mathematical proof isn’t just an intellectual exercise, it can tell us fundamental things about nature. 

Without a formal mathematical proof, we call something like Pythagoras’ Theorem a conjecture.

Professor De Gier says a conjecture in mathematics is a result that everyone believes to be true.

“But it hasn’t been proved logically in a rigorous sense,” he says.

“So, there may be a lot of numerical evidence and there may be strong and convincing arguments, but they are not establishing a truth beyond doubt.

“A great example is the Riemann Hypothesis about the zeros of the zeta function, which has been checked for the first 10,000,000,000,000 (ten trillion) cases. A proof that it is true for every case is still lacking and worth a million dollars,” says Professor De Gier.

“Proving it would shed light on many of the mysteries surrounding the distribution of prime numbers.”

“And occasionally something looks very convincing but then it’s shown, once you drill down to the fine detail, that it actually doesn’t hold and there may be exceptions.”

Wikipedia even has a category for ‘Disproved Conjectures’ – some, like Euler’s conjecture, stood for hundreds of years before being disproven.

Pythagoras didn’t invent the formula named after him, but came up with the first known proof. 

In the case of Pythagoras’ Theorem, however, the proof has been with us for thousands of years. In fact, Pythagoras didn’t invent the formula, it was known long before his time. He came up with the first known proof.

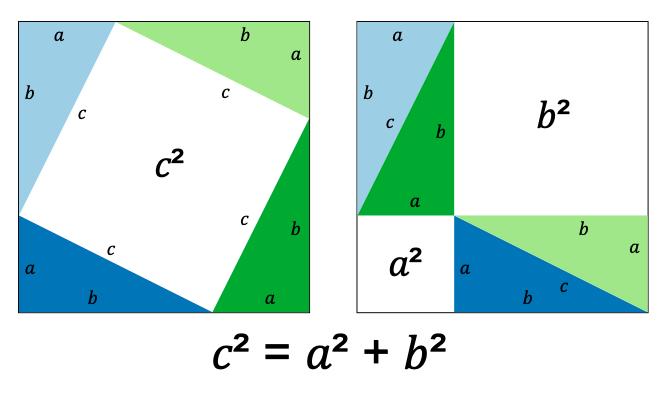
Pythagoras’ proof uses the indisputable fact that any right-angled triangle can be represented by two squares, one inside the other, with the corners of the inner square touching the edge of the outer.

The inner square has sides of length c (the actual length doesn’t matter because c can be any positive number), the outer square has length a+b, and the triangle it makes has side lengths a, b and c (as shown).

Changing the angle of the inner square changes the lengths of all three values.

Pythagoras showed that by rearranging the triangles inside the square, the white area, represented by c² in the above diagram, becomes two squares, one with area a² and one with area b². Hence, c² is always, no matter what dimensions you use, equal to a²+b².

Since Pythagoras, mathematicians through the ages have continued to find proofs of the theorem. In 1940, American mathematician Elisha Scott Loomis published a collection of proofs of Pythagoras’ Theorem.

The University of Melbourne team is no stranger to proofs.

The Australian Mathematical Society awarded the 2018 Gavin Brown Prize for best paper to Dr Beaton, Professor de Gier and Professor Guttman, along with Mireille Bousquet-Mélou from the Université de Bordeaux in France and Hugo Duminil-Copin from the Université de Genève in Switzerland, for a 2015 mathematical proof of the existence and critical surface tension for adsorption of polymers (long-chain molecules) in solution.

Professor Guttman, Professor de Gier and Dr Beaton were awarded the Gavin Brown Prize for best paper in 2018. 

The team used a mathematical representation of a polymer, called a ‘self-avoiding walk’, which are objects used in a branch of mathematical physics called statistical mechanics.

“A self-avoiding walk is a walk on a lattice – quite often a square lattice or a honeycomb lattice – where you can’t retrace any of the steps you’ve taken,” Professor Guttmann says.

“You can think of one walk as a single polymer, with random properties.”

Dr Beaton says that, often, finding a mathematical proof for a conjecture is a long, difficult process, involving trial-and-error, grunt work and the occasional eureka moment.

For Pythagoras, the eureka moment was the square-in-square representation of the triangle; for the Melbourne team and their colleagues, it was finding the best way to mathematically deal with the randomness.

“People tried a few things when it was first conjectured but no one made much headway, so it was clear a new idea was needed, but what that new idea should be was not obvious,” says Professor De Gier.

After following some dead-ends, the team focused in on a new idea in mathematics associated with lattice models, called ‘discrete holomorphicity’, which was popularised by Russian researcher Professor Stanislav Smirnov who won the Fields Medal for outstanding discoveries in mathematics in 2010.

Using this new kind of mathematics, the Melbourne team found that the honeycomb lattice was the right setting to prove their polymer problem.

Low dimensional topology: the mathematics of tangles and knots is explored at the Mathematical Research Institute. 

“For some reason, the mathematics of self-avoiding walks on a honeycomb lattice worked out nicely,” Professor de Gier says.

“If you want to do this on a square lattice, it doesn’t work, but for other problems, the square lattice would be better.”

Professor de Gier says that a mathematical proof isn’t just an intellectual exercise, it can tell us fundamental things about nature.

“Knowing the fact that something happens or where it happens is of interest, but having that logical reasoning is more interesting because it gives an insight into why things happen the way they do.”

This article was published by Pursuit.